Maths
>
A-Level
>
Algebra & Functions
>
What is pr...
What is proof by contradiction? How can x be both rational and irrational, please help
3 years ago
·
39 Replies
·
4208 views
Niko Lockman
39 Answers
Proof by contradiction is when you prove a statement wrong by showing that there is a flaw in the argument as there do exist numbers within the given range where the statement is false.
X is just a number, any number. Just as X can be even or odd, it can be rational, or irrational. Keep in mind it could be either rational or irrational, but cannot be both at the same time (just as a random number between 1 and 100 could be even or odd, but cannot be both).
Please let me know if you need me to clarify anything else,
Aaqib :)
Proof by contradiction is a form of proof, where a statement is made by the student which contradicts the statement proposed by the question. This is a form of proof since the opposite of the question is done and therefore an assumption has been made, the contradictory statement is eventually disproved to prove that the statement proposed in the question is correct
Hi Niko,
Proof by contradiction is a bit like when you and your friend order take out. When the food arrives in two identical bags, you look in one and see that it is not what you ordered. Instantly you know that the other bag must have your food in it (assuming the the process has not made a mistake). This is because you know that your food has to be in one of the bags, not in both and not in neither.
It is exactly the same with proof by contradiction. We know that x can't be both rational and irrational at the same time so we check to see whether it is rational. If it is rational, then it can't be irrational and if it is not rational, then it must be irrational!
One example might be proving by contradiction that the √2 is irrational.
First we would assume (pretend) √2 rational. If the √2 is rational, then we know that √2 can be expressed as a simplified fraction.
√2 = p/q
We can square both sides. (p^2 is the same as p squared)
2 = p^2 / q^2
Multiply by q^2 on both sides
2×q^2 = p^2
since any number times 2 is an even number, this tells us that p^2 is an even number.
If p^2 is an even number, then p must be an even number.
This means that we can write p as 2k and plug this back into the equation.
2×q^2 = (2k)^2
Expanding
2×q^2 = 4×k^2
Dividing by 2
q^2 = 2×k^2
This tells us that q^2 is an even number, meaning that q is also an even number. As q is an even number we can write q = 2n.
From using the equation at the top:
√2 = p/q = (2k)/(2n).
We assumed at the beginning that p/q is a simplified fraction, however (2k)/(2n) is not a simplified fraction since (2k)/(2n) = k/n. This is a contradiction.
Knowing that √2 is either rational or irrational, and that if √2 is rational it leads to a contradiction tells us that √2 must be irrational.
I hope this helps!
Proof by contradiction can only be used to validate a proposition as false.
The idea is to confront the proposition with contradiction and in this case, it is safe to conclude that the initial proposition is wrong.
For example:
Proposition A: Anna has no siblings.
If any evidence is presented showing that Anna has any number of siblings, we conclude that proposition A is wrong and we verify its validity by contradiction.
The contradiction: Anna has no siblings and a sibling at the same time.
(This proof is very useful because it is only necessary to have one contradictory situation to have a verdict. Therefore it is used in subjects like physics. )
In this context, no x can not be both rational and irrational.
Experienced maths tutor with masters in engineering
Hi Niko! A proof by contradiction does not directly prove something to be true, but rather proves that it is false. We can use it to show something else is true, though, if that's the only other option. One famous example that you are perhaps referring to in your question is used to show that the square root of two is an irrational number.
As you rightly point out, a number cannot be both rational and irrational – so to show that a number is irrational, we can instead show that it's definitely not rational. We can do that with a proof by contradiction: we start by assuming it is rational, then work through some equations based on that assumption until we prove two conclusions that cannot both be true. Then, if we've reached an impossible conclusion, we know that our initial assumption must have been wrong – the number can't be rational after all, so it must be irrational!
A proof by contradiction is any proof that works in this way – starting by assuming the opposite of what you are trying to prove, and showing that that assumption leads to an impossible (that is, self-contradictory) result.
I'm available for 1:1 private online tuition!
Click here to view my profile and arrange a free introduction.Cambridge Engineering Graduate with a love of Maths and Physics.
Proof by contradiction is one of the simpler methods of proof available. It essentially boils down to proving that two conditions cannot exist simultaneously assuming that a condition is true, working through what happens if that condition is true and showing that this leads to an impossible situation. Of course a chosen number cannot be rational and irrational, so if a proof had been conducted assuming that x is rational, and x is later found to be irrational, the situation we are describing is impossible.
I'm available for 1:1 private online tuition!
Click here to view my profile and arrange a free introduction.Experienced tutor of Maths, Further Maths and Science A-Level
To prove something by contradiction, you first assume the opposite is true (in this case, x is rational). If this leads to an impossible situation, or a contradiction, you know that your original statement must not be true.
For √2, we assume that it is first rational, and then we show that this assumption is not correct as it leads to a contradiction, meaning that it must be irrational.
I'm available for 1:1 private online tuition!
Click here to view my profile and arrange a free introduction.Proof by contradiction is a fundamental technique used in mathematics to prove a statement by assuming its opposite and showing that this assumption leads to a logical contradiction.
Here's how it works:
1. Start by assuming that the statement you want to prove is false.
2. Use this assumption to derive a series of logical conclusions.
3. Show that these conclusions lead to a contradiction, either with a known fact or with another assumption.
4. Conclude that the original assumption (i.e., the statement being false) must be incorrect, and therefore the statement must be true.
Now, let's apply this technique to the concept of rational and irrational number.
Think of it like a puzzle. You start with a statement, and then you try to build a logical argument around it. But if you assume the opposite is true, you'll eventually reach a point where the argument falls apart, and you'll have a contradiction. That's when you know that your original statement must be true.
Now, let's talk about rational and irrational numbers. A rational number is a number that can be expressed as a fraction, like 1/2 or 3/4. An irrational number, on the other hand, is a number that can't be expressed as a fraction, like the square root of 2 or pi.
So, can x be both rational and irrational? The answer is no. It's like trying to be in two places at once. A number is either rational or irrational, but it can't be both.
Imagine you have a number, x, and you try to prove that it's both rational and irrational. You start by assuming that x is rational, which means it can be expressed as a fraction. But then, you try to show that it's also irrational, which means it can't be expressed as a fraction. You'll eventually reach a point where you have a contradiction, because a number can't be both a fraction and not a fraction at the same time.
It's like trying to have a circle that's also a square. It just doesn't make sense. The two concepts are mutually exclusive, and you can't have both at the same time.
So, in the end, proof by contradiction helps us show that x can't be both rational and irrational. It's a powerful tool that helps us understand the nature of numbers and the world around us.
Now, let's consider the statement: "The square root of 2 is rational."
Assumption: Assume that the square root of 2 is rational, i.e., √2 = s/q, where a and b are integers and b is non-zero.
Derivation: If √2 = s/q, then we can square both sides to get:
2 = (s/q)²
2 = s²/q²
Multiplying both sides by b²: We get:
2q² = s²
Contradiction: This equation implies that s² is an even number (since it's equal to 2q²), which means that 's' must also be even (since the square of an odd number is always odd). Let's say s = 2k, where k is an integer.
Substituting s = 2k into the equation 2q² = s², we get:
2q² = (2k)²
2q² = 4k²
Dividing both sides by 2, we get:
q² = 2k²
This equation implies that q² is also even, which means that 'q' must also be even.
Hence, we started with the assumption that s/q is in its simplest form, i.e., that 's' and 'q' have no common factors. However, we've now shown that both 's' and 'q' are even, which means that they have a common factor of 2. This contradicts our original assumption!
Conclusion: Since our assumption that √2 is rational leads to a logical contradiction, we conclude that √2 must be irrational.
Proof by contradiction is a powerful logical technique used to prove the truth of a statement by assuming the opposite (the negation) of what you are trying to prove and showing that this assumption leads to a contradiction. If assuming the opposite leads to an impossibility or contradiction, then the original statement must be true.
Proof by contradiction works when there are only two options - an example being whether the square root of two is rational or irrational. The idea is that if we can show that one of the options is not true then the other one has to be true. So in the proof that the square root of two is irrational, we say that 'we suppose' that the the square root of two is rational, meaning we see what happens if it was. We then run into a 'contradiction'; two statements that follow from the assumption that the square root of two is rational, but cannot both be true at the same time. So then the square root of two cannot be rational and we must have written down something that was untrue somewhere along the line, which was supposing that root two was rational. If root two is not rational, it must be irrational. Note that at no point in this process have we said that root two is rational, we've just investigated what would happen if it was rational.
Hi Niko.
Proof by contradiction is a method of proof where instead of directly proving some statement, you show that if the statement were false then you hit a contradiction, which tells you that the statement cannot be false, so it must be true.
You are asking how x can be both irrational and rational. Well, it cannot be. A number is exactly one of rational or irrational, never both. One is the opposite of the other.
When proving by contradiction that some number x is irrational, we do indeed assume that it is rational. We are doing this in order to see that if x were rational by making logical deductions we can arrive at a contradiction, which tells us that the assumption that x is rational is false, and hence x is not rational, i.e. x is irrational.
As an example, consider proving that √2 is irrational. Here is how the proof goes.
Assume that √2 is rational. Then it can be written as a fraction, so let √2 = a/b where a and b have no common factors other than 1 (this just means that the fraction is written in simplest form, something that we can do to any fraction).
Now squaring both sides we have 2 = (a/b)^2 = a^2/b^2.
Multiplying by b^2 we have 2b^2 = a^2. Thus a^2 is even, since it equals 2 times some integer.
Therefore a must be even (the square of an even is even, and the square of an odd is odd. think about why this is). We can write a = 2k for some integer k since a is even.
We then have 2b^2 = (2k)^2 = 2^2 k^2 = 4k^2 and dividing by 2 we have b^2 = 2k^2, so b^2 is even and hence b is even.
But then both a and b are even which means the fraction is not in simplest form since we assumed they have no common factors other than 1. This is a contradiction. Therefore √2 is not rational.
Hence √2 is irrational.
Hope this helps!
Hey Niko! Basically with a proof of contradiction you start with the original statement, lets say "The square root of 2 is irrational". you start by taking the CONTRADICTION of that, meaning you are going to (try to) prove that the square root of 2 is actually rational. When you try to solve this, you will in fact turn out with the conclusion that the square root of 2 cannot be rationally written down, contradicting your statement of root 2 being rational, hence the original statement of it being irrational is true! Here is a picture, see if this helps!
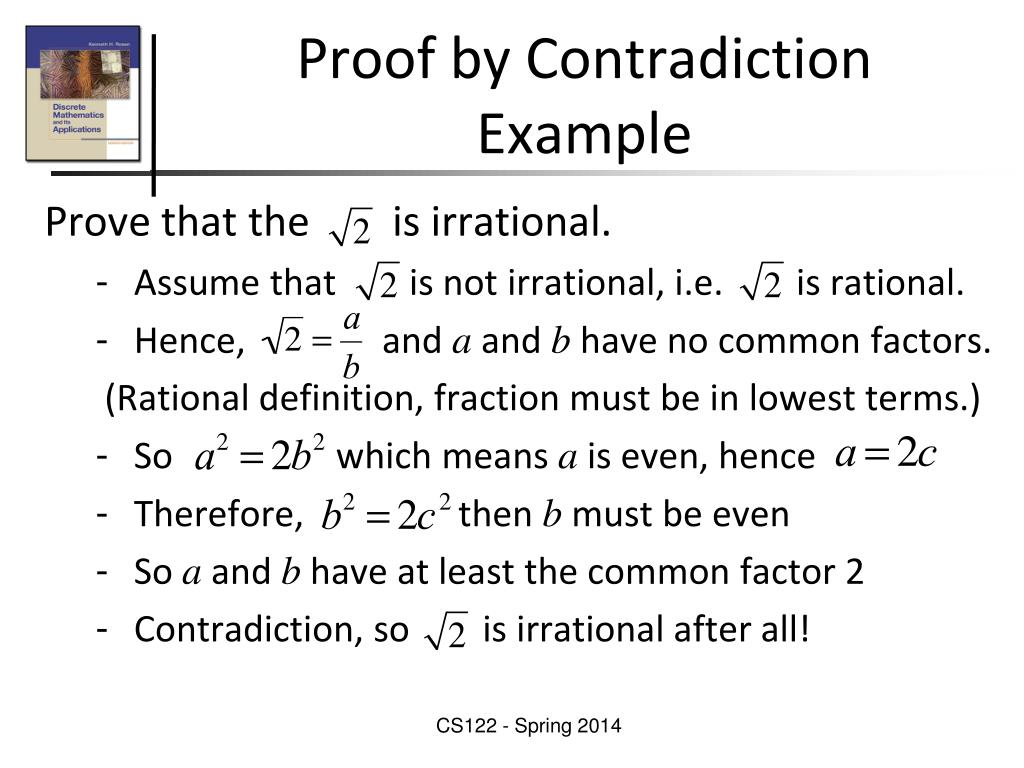
Proof by contradiction works by assuming some mathematical statement to be false, but then after following mathematically logical steps, one reaches a conclusion that we know to be false. This means that, since our intermediate steps in the proof were all true, our original assumption must be incorrect - the original statement must be true!
Regarding rational and irrational numbers; a rational number cannot also be an irrational number. The way that irrational numbers can be defined is as a real number that is not rational.
Hope that helps :)
There is no substitution for trying your best and working hard.
6 reviews
Proof by contradiction is when you assume the opposite of what you are trying to prove, (e.g. if you are trying to prove that root(2) is irrational, you will assume it is a rational number as your first step) and then through a correct process produce a situation which is impossible or incorrect, meaning your initial statement must have been initially incorrect and so the opposite must be true. It is not possible for x to be both rational and irrational, we will assume one, show this is impossible and hence prove it must be the other.
I'm available for 1:1 private online tuition!
Click here to view my profile and arrange a free introduction.Experienced and enthusiastic A-level/ GCSE/ Degree Maths and Economics tutor
14 reviews
Proof by contradiction is a method of proving where you first assume your statement to be false. Then use logical steps to show that indeed the statement is true. As a statement can not be true and false at the same time, we deduce that the statement is true. A classic example is proving that the square root of 2 is irrational. So first you assume it to be rational and use logical steps to deduce that actually, it can't be rational. Therefore we conclude that it is irrational.
I'm available for 1:1 private online tuition!
Click here to view my profile and arrange a free introduction.Think you can help?

Need an A-Level Maths tutor?
Get started with a free online introductions with an experienced and qualified online tutor on Sherpa.
Find an A-Level Maths Tutor